“building blocks”- 2 words I grew up with. be it LEGOs, MECHANO sets, or even sand castles-it all is based on building blocks. Brick is a great example of a building block- a single piece of material that is modular, infinitely repeatable, and structurally stable. Another great example in this digital world is material mapping and 3D shape draping-tessellation. the art of using a 2D plane/shape to generate a 3D object. in the virtual world, we are all too familiar with the use of triangles to generate any shape.
I digress. it all started with a simple question-why are paver tiles so predictably similar in shape. with that question started the quest for the math behind the paver, which finally resulted in a unique shape (or 2) that I love. the writeup below is some fragments of that research focussed on the pentagon-shaped tile.
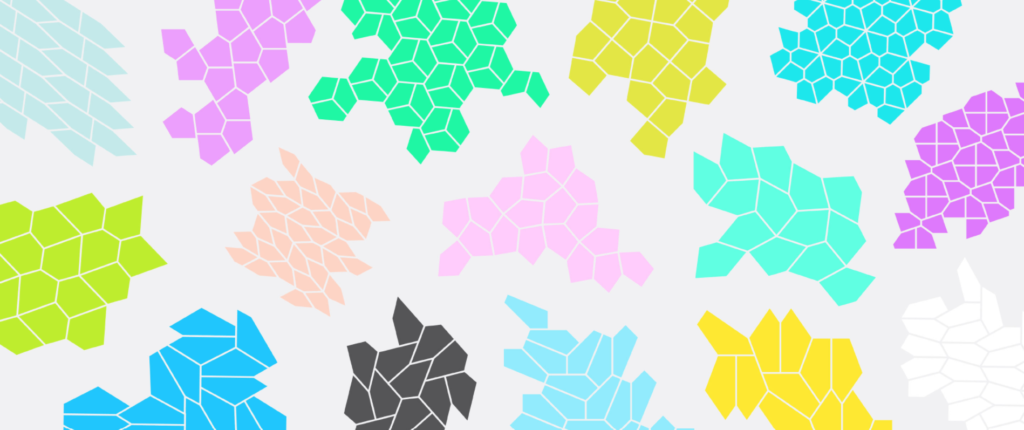
tiling and use of just one shape-monohedral tiling is a tessellation in which all tiles are congruent; it has only one prototile. monohedral tiling is a great way to make paver tiles as it allows for a single mold to be used. this translates into 3 main shapes-triangle, square, and a hexagon-and hence the limited number of shapes. I intentionally left out the pentagon. A regular pentagonal tiling on the Euclidean plane is impossible because the internal angle of a regular pentagon, 108°, is not a divisor of 360°, the angle measure of a whole turn. Unlike the other shapes I mentioned, pentagonal tiling is more complex and nuanced-so much so it took around 100 years to figure it out…and there are 15 types of pentagonal monohedral tiling…so far.
The first five were discovered during investigations of German mathematician Karl Reinhardt in 1918. After a gap of 50 years, R. B. Kershner found three more in 1968. Richard James subsequently discovered a ninth type of pentagonal tiling in 1975 and over the next few years, Marjorie Rice discovered another four types. Rolf Stein found a 14th tiling in 1985. The most recently discovered 15th tiling was found by Casey Mann, Jennifer McLoud and David Von Derau of the University of Washington Bothell in 2015 using a computer to exhaustively search through a large but finite set of possibilities (Bellos 2015).
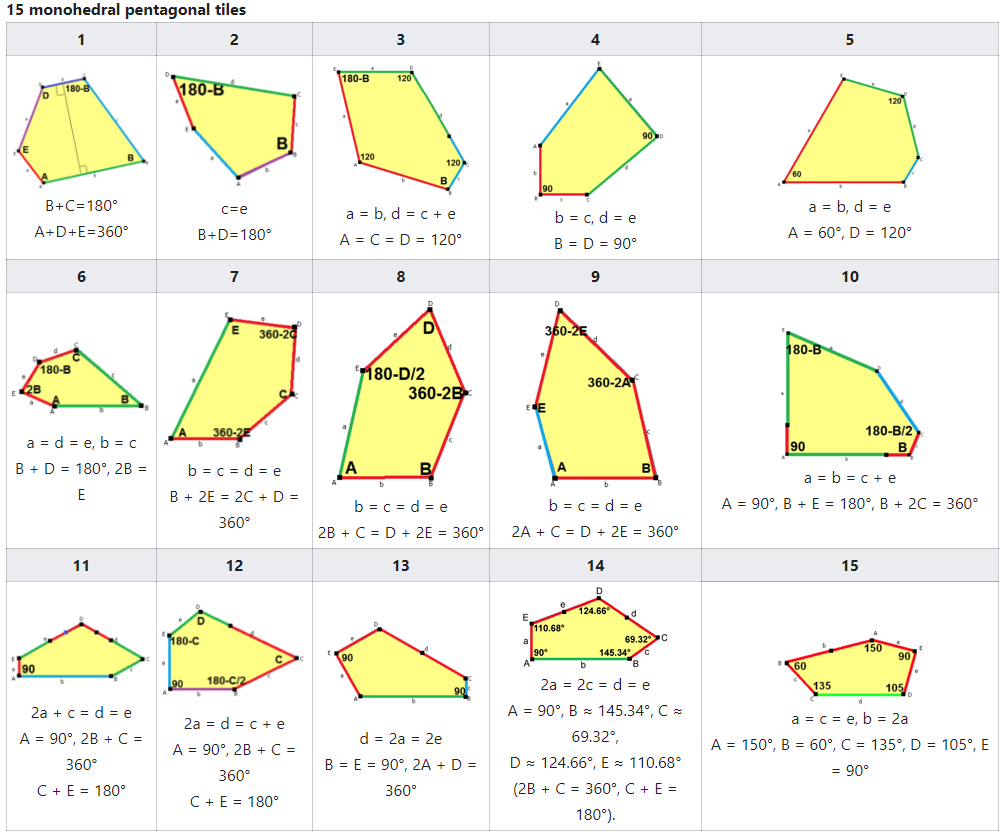
It has not been proven whether these 15 cases exhaust all possible tilings, but no others are known.
Michaël Rao, a 37-year-old mathematician at CNRS (France’s national center for scientific research) and the École Normale Supérieure de Lyon, finally completes the classification of convex polygons that tile the plane by conquering the last holdouts: pentagons, which have resisted sorting for 99 years
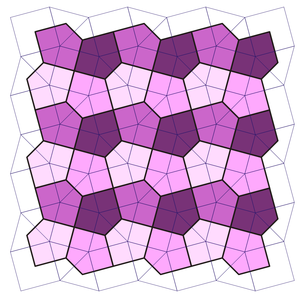
so, with all that said, i am going to focus on type 4 and type 15.
I love type 4 because the unique shape is a derivative of a simpler shape (the hexagon). Its also called the Cairo pentagonal tiling. it is at once both a pentagon and a hexagon, and it varies based on where and how you look at it-as an architect, having that as a design feature is just perfect!

in fact, it can also be split into (2) squares and (3) equilateral triangles.
I looked around for applications, and I saw this youtube video of a DIY paver solution that integrates function and form together-and I had to include this-truly inspirational-especially the part about stacking the pavers to create vertical interest.

techo-bloc makes a hexagonal tile that has the same proportions of (4) pentagonal tiles

So, let’s talk about type 15-the youngest of the pentagonal tiling set so far. I tried to draft the tile and the pattern on CAD, and it was complicated. I can understand why people stick to squares and rectangles. Type 15 can be quite restrictive.
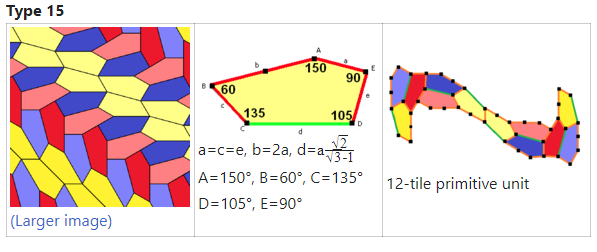
so what is the allure of type 15, you ask! of the many paver tiles I have had the chance to research, very few are “directional”-the “field” is not meant to be directional. However, I love to be able to spec and design directional paver tiles that can evoke a sense of movement without compromising field neutrality and structure. Most importantly, the arrangement needs to look random, yet there needs to be a repeating pattern. paver manufacturers are beginning to realize the need for this with the introduction of longer rectilinear tiles that evoke directionality-at the cost of being field “neutral”. the other disadvantage is that as the proportions become more linear, it comes at the cost of strength-the shorter the width relative to the length, the more likely the tile will break.
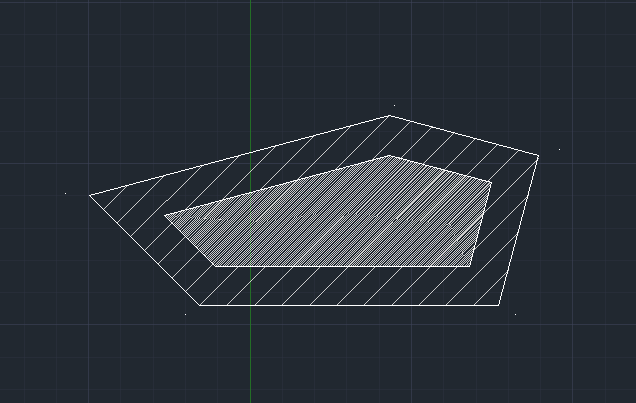
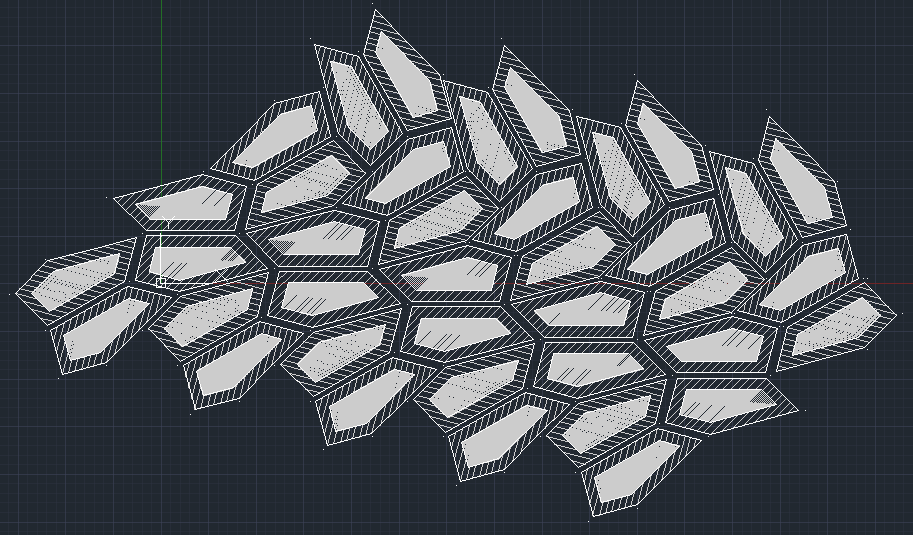
Enter type 15-a tile that incorporates the linear proportions without compromising strength. it provides direction, yet managing to be field neutral.
a case study was documented in the magazine-landscape architecture December 2018 print. a team from Sasaki led by Courtney Goode studied this particular type and studied prototypes, created molds as well as built a batch and installed it.


I really hope this becomes mainstream, or I can get my hands on a few hundred of these for my patio